Quadratic Equations

After studying this section, you will be able to:

solve quadratic equations by factorising
solve quadratic equations by completing the square
solve quadratic equations by using the formula
solve simultaneous equations when one of them is quadratic

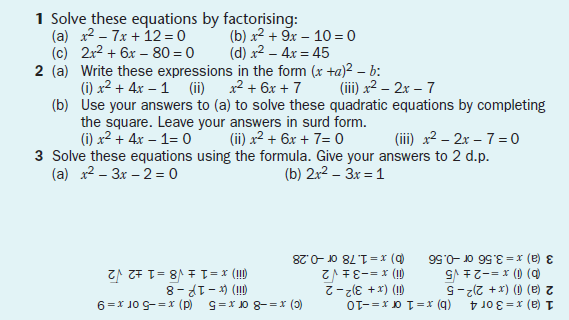
Solving quadratic equations by factorising

Unless a graphical method is asked for, quadratic equations on the non-calculator paper will probably involve factorising or completion of the square. Quadratic equations can have two different solutions or roots.

You may need a quick look at 'factorising' again to remind yourself how to factorise expressions such as:

x2 − x − 6

which factorises into (x − 3)(x + 2),

a^₂ − 3a

which factorises into a(a − 3)

and

b² − 2b + 1

which will factorise into (b − 1)².

This video shows you how to solve a quadratic equation by factoring

Solving quadratic equations by completing the square

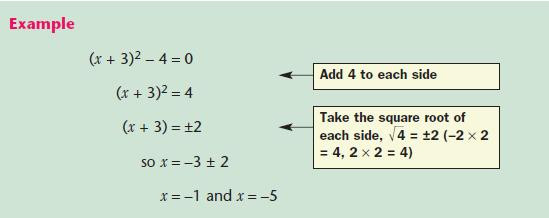
NOTE: Check by substituting both roots back into the original equation.

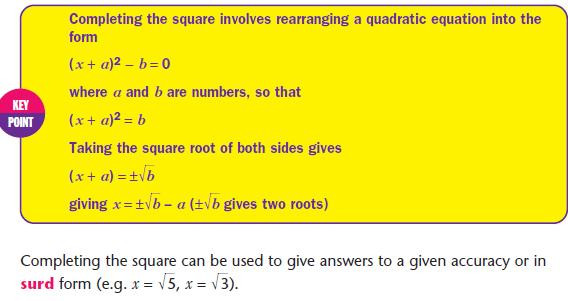
This following is a common way to lead into asking you to use completion of the square.

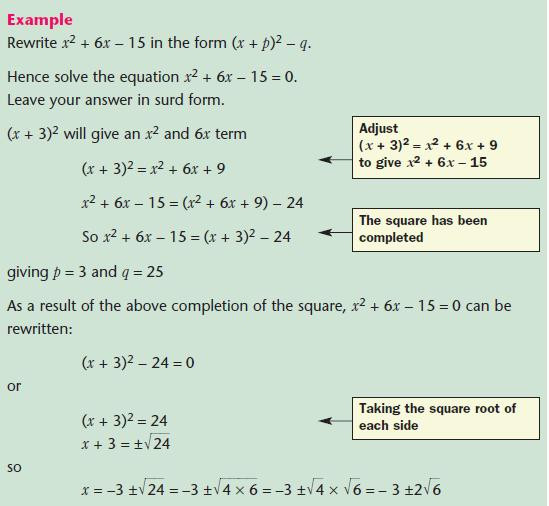
NOTE: Remember in, for example, (x + n)² the number of xs (called the coefficient of x) is 2_n. So the coefficient of x will be 6 in (x + 3)².

Solving quadratic equations by using the formula

When using the quadratic formula, don’t forget the ‘2a’denominator. Also, be careful when dealing with negative numbers

inside the square root. State your values of a,b and c to be used in the formula.

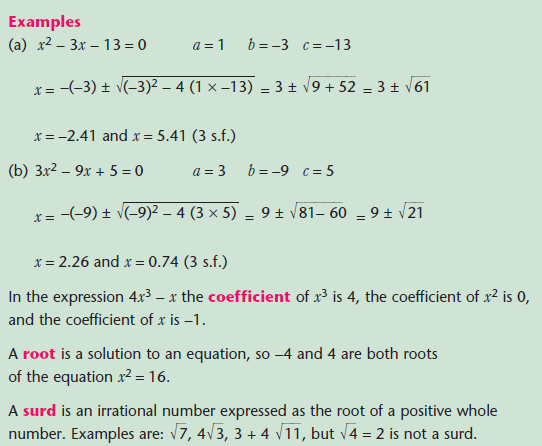
NOTE: The above calculations are easily checked − especially if your calculator can store numbers as variables.

PROGRESS TEST

Log in